
The trip described can be to a ultracompact neutron star with any given mass (and hence Schwarzschild radius), and therefore, as in the previous sections, all distances will first be given in terms of the Schwarzschild radius, and later, in parenthesis, in terms of kilometers for a specific model. For the canonical specific model, a star will again be used with mass 1.4 M_sun, but this time with a surface of R_* = 4/3 R_S, the minimum allowed without violating the dominant energy condition. [30] More specifically, this hypothetical star has R_S = 4.2 km and R_* = 5.6 km.
Any object compact enough to have a photon sphere will always appear to have the apparent size of its photon sphere. [31] This is because photons coming to the viewer from the object's limb must have orbited near the photon sphere before escaping to be seen. Therefore, one cannot measure the actual stellar radius of such an object by measuring the angular radius seen. Were the star to shrink the angular radius would appear to be the same. One cannot even measure a decrease in R_* by noting the change in the positions of background stellar images, because there will be no change. The only change the observer could note is that of increased apparent distortion of surface features.

The hypothetical journey begins 100 R_S (420 km) from the neutron star. Fig. 3a shows the distortion effects a viewer would see from this distance. The distortions of the background sky are precisely the same as in the black hole description in the previous section. Essentially an undistorted night sky is visible from the viewer's location with a small patch of barely resolved fuzz in the center. The constellation Orion is clearly visible to the right of center.



As the viewer nears the ultracompact neutron star the fuzzy patch breaks up to become a conglomeration of secondary images. This is shown in the succession of Figs. 3b, 3c, and 3d. Fig. 3b shows the neutron star from a distance of 50 R_S (210 km). Fig. 3c shows the neutron star from a distance of 25 R_S (105 km), while Fig. 3d shows the neutron star from a distance of 10 R_S (42 km).
There are several interesting aspects of Fig. 3d. Again, the positions of the stellar images are exactly the same as if the lens were a black hole. The blueshifting of the image colors would also be exactly the same. The first sky Einstein ring has been drawn in, and the primary and secondary images of Sirius and the belt of Orion are labelled. The whole surface is visible, and some portions can even be seen to have a second image just inside the apparent position of the photon sphere. The first surface Einstein ring can be seen to have about 95 percent of the radius of the apparent position of the photon sphere in Fig. 3d. A complete image of the whole surface of the neutron star is visible inside this first surface Einstein ring. Another `mirror written' and greatly distorted version of the entire surface would be seen in the annular space between the first and second surface Einstein rings (nearer the limb). Higher order sets of images are not shown but would be difficult to see as they would occupy even thinner annular rings seen extremely close to the photon sphere limb.
A surface feature near the limb of the neutron star would have the same surface brightness as an identical surface feature near the center, as seen by any observer. This is because there would be no limb darkening observable: the limb of the image of the neutron star surface appears equally as bright as the central regions. This is again a result of the conservation of surface brightness and that the surface emission is assumed isotropic.






The viewer now does an orbit around the neutron star at the radius of 10 R_S (42 km). The distortions the viewer would see are shown in Figs. 3d - 3j. These figures depict viewing angles for relative angular positions of 0 degrees, 5 degrees, 10 degrees, 90 degrees, 180 degrees, 270 degrees, and 360 degrees around the orbit. A complete orbit would encompass, of course, 360 degrees and so Fig. 3j is the same as Fig. 3d.
Figs. 3e and 3f, showing a relative 5 degrees and 10 degrees angle compared to Fig. 3d, shows revealing differences when compared with Fig. 3d. The comparison allows the reader to discern the first sky Einstein ring fairly easily. As before, the differences in the stellar positions are discussed in the above section on black holes. Comparison of the changes in the apparent positions of the surface features are quite similar but harder to discern in the figures.

The viewer is now taken even closer to the neutron star. Fig. 3k shows the distortions from 3 R_S (12.6 km): at twice the height of the photon sphere. The viewer is still looking directly at the neutron star.



Figs. 3l, 3m, and 3n show distortions from 1.5 R_S (6.3 km), the height of the photon sphere, as the viewer's inspection angle pans up. The relative angles the viewer is looking with respect the direction of the neutron star are 30 degrees, 60 degrees, and 90 degrees, respectively.
In Fig. 3n, the viewer now looks directly along the photon sphere. The self Einstein ring where viewers could see the backs of their heads is the photon sphere horizon line dividing the land and the sky. In exact accordance with the black hole case (Figs. 2l, 2m, and 2n), Fig. 3n shows the first sky Einstein ring would be an invisible line about 2/9 ths of the way toward the top of the plot above the photon sphere. Similarly, the first surface Einstein ring could be drawn in as a line just under (about a line's width below) the photon sphere.
The apparent color of the surface features would now appear less redshifted. This is because the light no longer has to climb out of so deep a potential well to reach the observer. The surface would still appear redshifted to some degree, as the light must climb from the surface only to the photon sphere, not nearly so much as before.



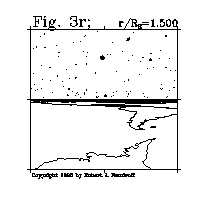

The viewer now does an orbit at the height of the photon sphere, as shown in Figs. 3n - 3s. Fig. 3o shows the view 5 degrees from the Fig. 3n into the orbit, while Figs. 3p, 3q, 3r, and 3s are 90 degrees, 180 degrees, 270 degrees, and 360 degrees, respectively. As the viewer moves along the photon sphere, both the stellar images and the surface images appear to move in peculiar ways. Stars that would have appeared behind the observer in the absence of large gravitational light deflection effects now have images that appear in front of the viewer and above the photon sphere, but below the first sky Einstein ring. A star that approaches the opposite side of the neutron star from the viewer appears to approach the first Einstein ring from below, as this secondary image get brighter. As the motion of the viewer causes the star to move relatively from behind to in front of the viewer (crossing close to the opposite side of the neutron star), the secondary stellar image moves much faster, reaches it maximum brightness, and moves quickly (below the first sky Einstein ring) out of the picture. Shortly thereafter, the brighter primary stellar image above the first sky Einstein ring comes into view on the opposite side of the plot. As the ship moves further around in its orbit, this primary stellar image rises and dims.
Similarly, surface features actually just behind the viewer are visible well in front of the viewer just below the photon sphere but above the first surface Einstein ring.


The viewer now descends to the neutron star surface. This is shown in Figs. 3t and 3u. Fig. 3t shows the surface distortions from a height of 1.4 R_S (5.88 km) and Fig. 3u illustrates the distortions visible from just above the surface, at a height of 1.33 R_S (5.6 km).
The viewer now appears to be in a slight bowl. Looking horizontally the viewer does not see the sky but rather the surface. Even looking at an angle slightly up, the viewer would be observing the surface, no matter which azimuthal direction is observed. This is because of the photon orbits that are trapped. Some photon orbits leave the neutron star and fall back, never reaching infinity. If the viewer looks along these paths, the viewer will be looking slightly up and still seeing an image of the surface. In fact the sky now appears scrunched up to occupy a smaller "hole" above the observer.
As before, the apparent line dividing land and sky still marks the apparent position of the photon sphere. Also, as before, when orbiting at the photon sphere, the sky Einstein rings are all seen above the apparent photon sphere position. The first sky Einstein ring is again about 1/9 th of the length of the plot border above the photon sphere. The many surface Einstein rings and complete images of the stellar surface are contained just below the photon sphere, although they together occupy only a thin sliver below the photon sphere.



In the last sequence, the viewer pans around to see the whole neutron star surface. This is shown in Figs. 3v - 3w, showing the surface image distortions at relative viewing angles of 120 degrees, and 240 degrees. The entire surface and sky is visible, but not in a single field of view. Lastly, Fig. 3x has the viewer looking along the original northward direction, the same as Fig. 3u.
The type of map projections the Schwarzschild metric creates are different than any commonly used type of map projection, [32] and different than any known type of map projection that the author is aware of.
A video has been produced featuring these lens effects [33] and is available free of charge. To receive a copy of the VHS tape, please write to the author's address given under the paper's title. The author will try to maintain an abundance of copies of the video on the latest popular video display medium (be it HDTV tape, laser disc, etc.) to service requests even several years after this article's publication.